Q 1 – ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the areas of triangles ABC and BDE is:
(A) 2:1
(B) 1:2
(C) 4:1
(D) 1:4
Ans. Option (C) is correct.
Q 2 – Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio :
(A) 2 : 3
(B) 4 : 9
(C) 81 : 16
(D) 16 : 81
Ans. Option (D) is correct.
Q 3 – In the figure given below,∠ BAC = 90° and AD BC. Then :
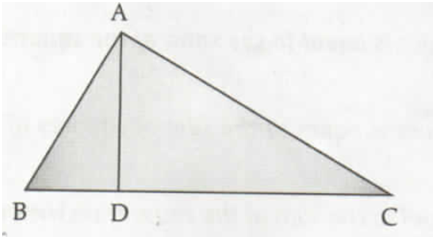
(A) BD x CD = BC2
(B) AB x AC = BC2
(C) BD x CD = AD2
(D) AB x AC = AD2
Ans. Option (C) is correct
Q 4 – If ∆ABC ~ ∆EDF and ∆ABC is not similar to ∆DEF, then which of the following is not true?
(A) BC x EF = AC x FD •
(B) AB x EF = AC x DE
(C) BC x DE = AB x EF
(D) BC x DE = AB x FD
Ans. Option (C) is correct.
Q 5 – If in two triangles ABC and PQR, ![]() =
= ![]() =
= ![]() then :
then :
(A) ∆PQR ~ ∆CAB
(B) ∆PQR ~ ∆ABC
(C) ∆CBA ~ ∆PQR
(D) ∆BCA ~ ∆PQR
Ans. Option (A) is correct
Q 6 – In triangles ABC and DEF, ∠B = ∠E, ∠F =∠C and AB = 3DE. Then, the two triangles are:
(A) congruent but not similar
(B) similar but not congruent
(C) neither congruent nor similar
(D) congruent as well as similar
Ans. Option (B) is correct.
Q 7 – Directions: In the following questions, A statement of Assertion is followed by a statement of Reason (R). Mark the correct choice as.
(A) Both A and R are true and R is the correct explanation of A
(B) Both A and R are true but R is NOT the correct explanation of A
(C) A is true but R is false
(D) A is false and R is True
Q 8 – Assertion (A): If in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangle, then triangles will be similar.
Reason (R): If the ratio of the corresponding altitudes of two similar triangles is ![]() then the ratio of their areas is
then the ratio of their areas is ![]()
Ans. option (C) is Correct.
Q 9 – Assertion (A): If D is a point on side QR of ∆PQR such that PD L QR, then ∆PQD ∆RPD.
Reason (R): In the figure given below, if ∠D = ∠C then ∆ADE ∆ACB
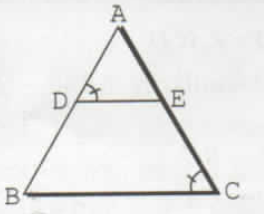
Ans. Option (D) is Correct.
Q 10 – Assertion (A): Corresponding sides of two similar triangles are in the ratio of 2 : 3. If the area of the smaller triangle is 48 cm2, then the area of the larger triangle is 108 cm2.
Reason (R): If D is a point on the side BC of, a triangle ABC such that ∠ADC = ∠BAC, then CA2 = CB x CD.
Ans. Option (B) is correct.
Q 11 – Assertion (A): In an equilateral triangle of side 3√3 cm, then the length of the altitude is 4.5 cm
Reason (R): If a ladder 10 cm long reaches a window 8 m above the ground, then the distance of the foot of the ladder from the base of the wall is 6 m.
Ans. Option (B) is correct.
Attempt any four Sub -part from each question.
Each Sub -part Carries 1 mark
Read the following text and answer the following questions on the basis of the same.
SCALE FACTOR AND SIMILARITY
SCALE FACTOR
A scale drawing of an object is of the same shape as the object but of a different size. The scale of a drawing is a comparison of the lengthused on a drawing to the length it represents.
The value of scale is written as a ratio.
SIMILAR FIGURES
The ratio of two corresponding sides in similar figures is called the scale factor
Scale factor = ![]()
If one shape can become another using Resizing then the shapes are Similar.
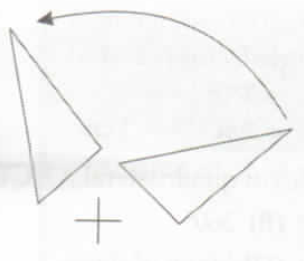
Hence, two shapes are Similar when one can become the other after a resize, flip, slide or turn.
Q 12 – A model of a boat is made on the scale of 1 : 4. The model is 120 cm long. The full size of the boat has a width of 60 cm. What is the width of the scale model ?

(A) 20 cm
(B) 25 cm
(C) 15 cm
(D) 240 cm
Q 13 – What will effect the similarity of any two polygons ?
(A) They are flipped horizontally
(B) They are dilated by a scale factor
(C) They are translated down
(D) They are not the mirror image of on another.
Q 14 – If two similar triangles have a scale factor of a : b, which statement regarding the two triangles is true ?
(A) The ratio of their perimeters is 3a : b
(B) Their altitudes have a ratio a : b
(C) Their medians have a ratio [latex]\frac{a}{2}[/latex}:b
(D) Their angle bisectors have a ratio a2 : b2
Q 15 – Below you see a student’s mathematical model of a farmhouse roof with measurements. The attic floor, ABCD in the model, is a square. The beams that support the roof are the edge of a rectangular prism, EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the `middle of CT, and H is the middle of DT. AIl the edges of the pyramid in the model have length of 12m

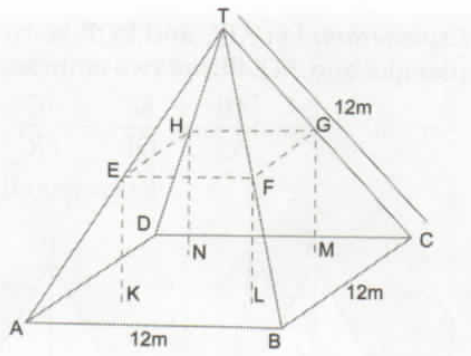
What is the length of EF, where EF is one of the horizontal edges of the block ?
(A) 24 m
(B) 3 m
(C) 6 m
(D) 10 m
Ans. Option (C) is Correct.
Read the following text and answer the below questions:
Seema placed a light bulb at point O on the ceiling and directly below it placed a table. Now, she put a cardboard of shape ABCD between table and lighted bulb. Then a shadow of ABCD is casted on the table as A’B’C’D’ (see figure). Quadrilateral A’B’CD’ in an enlargement of ABCD with scale factor 1 : 2, Also, AB = 1.5 cm, BC = 25 cm, CD = 2.4 cm and AD = 2.1 cm; ∠A = 105°, ∠B = |100°, ∠C = 70° and ∠D = 85°
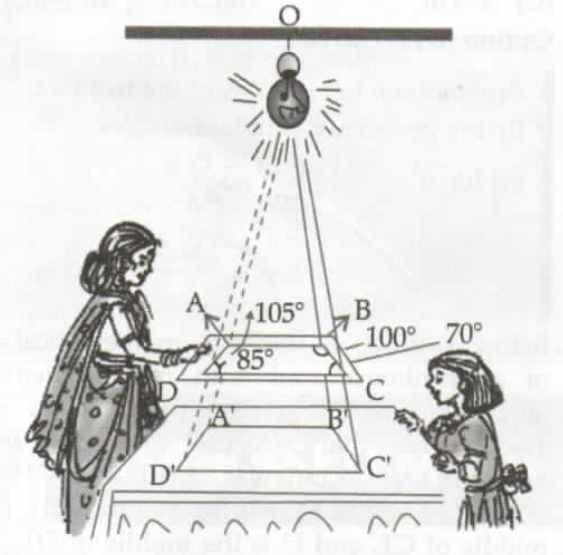
Q 16 – What is the measurement of angle A’ ?
(A) 105°
(C) 70°
(B) 100°
(D) 80°
Ans. option (A) is Correct.
Q 17 – What is the sum of angles of quadrilateral A’B’CD’ ?
(A) 180°
(B) 360°
(C) 270°
(D) None of these
Ans. Option (B) is correct.
Q 18 – What is the ratio of sides A’B’ and AD’ ?
(A) 5:7
(B) 7:5
(C) 1:1
(D) 1:2
Ans. Option (A) is correct.
Read the following text and answer the below question
SIMILAR TRIANGLES
Vljay is trying to find the average height of a tower near his house. He is using the properties of Similar triangles. The height o£ Vijay’s house if 20 m when Vijay’s house casts a shadow 10 m long on the ground. At the same time, the tower casts a shadow 50 m long on the ground and the house of Ajay casts 20 m shadow on the ground.
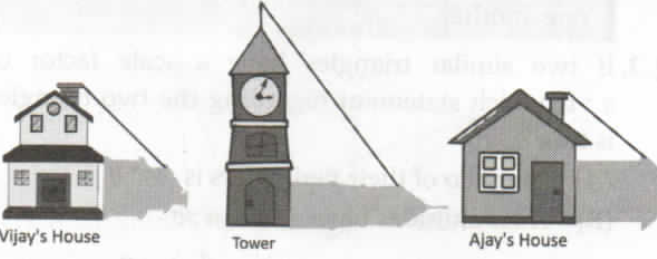
Q 19 – What is the height of the tower?
(A) 20 m
(B) 50 m
(C) 100 m
(D) 200 m
Ans. Option (C) is correct
Q 20 – What will be the length of the shadow of the tower when Vijay’s house casts a shadow of 12 m?
(A) 75 m
(B) 50 m
(C) 45 m
(D) 60 m
Ans. Option (D) is correct.
Q 21 –What is the height of Ajay’s house?
(A) 30 m
(B) 40 m
(C) 50 m
(D) 20 m
Ans. Option (B) is correct
Q 22 – When the tower casts a shadow of 40 m, same time what will be the length of the shadow of Ajay’s house?
(A) 16 m
(B) 32 m
(C) 20 m
(D) 8 m
Ans. Option (A) is correct.
Q 23 – When the tower casts a shadow of 40 m, same time what will be the length of the shadow of Vijay’s house?
(A) 15 m
(B) 32 m
(C) 16 m
(D) 8 m
Ans. Option (D) is correct.
Read the following text and answer the below questions:
Rohan wants to measure the distance of a pond during the visit to his native. He marks points A and 8 on the opposite edges of a pond as shown in the figure below. To find the distance between the points, he makes a right-angled triangle using rope connecting\B with another point C are a distance of 12 m, connecting C to point D at a distance of 40 m from point C and the connecting D to the point A which is are a distance of 30 in from D such the ∠ADC = 90°.
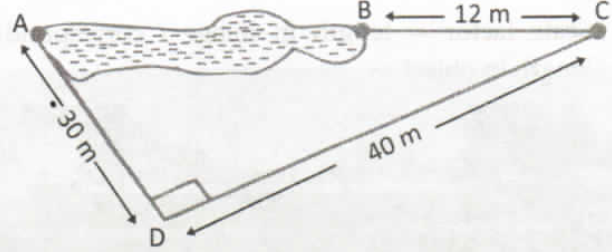
Q 24 – Which property of geometry Will be Used to find the distance Ac?
(A) Similarity of triangles
(B) Males Theorem
(C) Pythagoras Theorem
(D) Area of similar triangles
Ans. Option (C) is correct
Q 25 – What is the distance AC?
(A) 50 m
(B) 12 m
(C) 100 m
(D) 70 m
Ans. Option (A) is correct.
Q 26 – Which is the following does not form a Pythagoras triplet?
(A) (7,24,25)
(B) (15,8,17)
(C) (5,12,13)
(D) (21,20, 28)
Ans. Option (D) is correct
Q 27 – Find the length AB?
(A) 12 m
(B) 38 m
(C) 50 m
(D) 100 m
Ans. Option (B) is correct
Q 28 – Find the length of the rope used.
(A) 120 m
(B) 70 m
(C) 82 m
(D) 22 m
Ans. Option (C) is correct.
Read the following text and answer the below questions:
SCALE FACTOR
A scale drawing of an object is the same shape at the object but a different size. The scale of a drawing is a comparison of the length used on a drawing to the length it represents. The scale is written as a ratio. The ratio of two corresponding sides in similar figures is called the scale factor
Scale factor =length in image/corresponding length in Object.

If one shape can become another using revising, then the shapes are similar. Hence, two shapes are similar when one can become the other after a resize, flip, slide or turn. In the photograph below showing the side view of a train engine .Scale factor is 1:200
This means that a length of 1 cm on the photograph above corresponds to a length of 200 cm or 2 in, of the actual engine. The scale can also be written as the ratio of two lengths.
Q 29 – If the length of the model is 11 cm, then the overall length of the engine in the photograph above, including the couplings(mechanism used to connect) is:
(A) 22 an
(B) 220 cm
(C) 220 m
(D) 22 m
Ans. Option (A) is correct
Q 30 – What will affect the similarity of any two polygons?
(A) They are flipped horizontally
(B) They are dilated by a scale factor
(C) They are translated down
(D) They are not the mirror image of one another.
Ans. Option (D) is correct.
Q 31 – What is the actual width of the door if the width of the door in photograph is 0.35 cm?
(A) 0.7 m
(B) 0.7 cm
(C) 0.07 cm
(D) 0.07 m
Ans. Option (A) is correct
Q 32 – If two similar triangles have a scale factor 5:3 which statement regarding the two triangles is true?
(A) The ratio of their perimeters is 15:1
(B) Their medians have a ratio 10:4
(C) Their angle bisectors have a ratio 11:5
(D) Their altitudes have a ratio 25:15
Ans. Option (B) is correct.
Q 33 – The length of AB in the given figure:
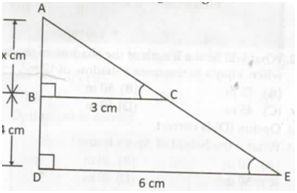
(A) (8) cm
(B) (6) cm
(C) (4) cm
(D) (10) cm
Ans. option (C) is Correct.
Q 34 –In the figure given below, two line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to : A
(A) 50°
(B) 30°
(C) 60°
(D) 100°
Ans. Option (D) is correct.
Q 35 – The shadow of a stick 5 in long is 2 in. At the same time the shadow of a tree 12.5 in high is
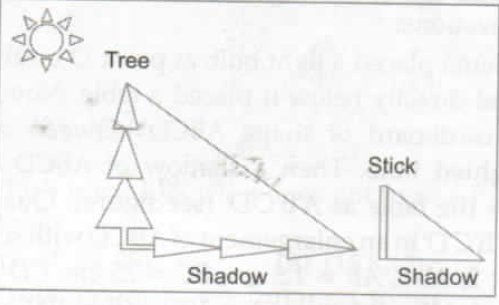
(A) 3m
(B) 3.5
(C) 4.5
(D) 5 m
Ans. option (D) is Correct.