NCERT SOLUTIONS FOR CLASS 10 MATHS PAIR OF LINEAR EQUATIONS IN TWO VARIABLES CHAPTER 3 Ex 3.1

Pair of Linear Equations in Two Variables Ex 3.1

Question 1.
Aftab tells his daughter, “Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be”. Isn’t this interesting? Represent this situation algebraically and graphically.
Solution:

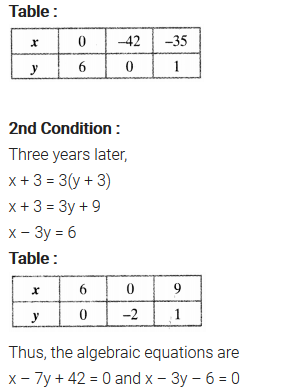
Question 2.
The coach of a cricket team buys 3 bats and 6 balls for ₹ 3900. Later, she buys another bat and 3 more balls of the same kind for ₹1300. Represent this situation algebraically and geometrically.
Solution:

Let us assume that the cost of a bat be ‘Rs x’
And, the cost of a ball be ‘Rs y’
According to the question, the algebraic representation is
3x+6y = 3900
And x+3y = 1300
For, 3x+6y = 3900
Or x = (3900-6y)/3
The solution table is
For, x+3y = 1300
Or x = 1300-3y
The solution table is
The graphical representation is as follows.

Thus, we have the following table of points.

x	100	400	700
y	400	300	200

Plotting the points of each table, we obtain the required graph of two intersecting lines.

Question 3.
The cost of 2 kg of apples and 1 kg of grapes on a day was found to be ₹160.  After a month, the cost of 4 kg of apples and 2 kg of grapes is ₹300. Represent the situation algebraically and geometrically.
Solution:

Let Cost of one Kg of apples = Rs. x and the cost of one Kg of grapes = Rs. y

A.T.Q.

1st Condition:

2x + y = 160

Table:

x	80	60	40
y	0	40	80

2nd Condition:

4x + 2y = 300

Table

x	60	40	20
y	30	70	110

Thus, algebric situations are 2x + y = 160 and 4x + 2y = 300

Class 10 Maths Pair of Linear Equations in Two Variables Mind Map

System of a Pair of Linear Equations in Two Variables

An equations of the form Ax + By + C = 0 is called a linear equation in two variables x and y where A, B, C are real numbers.
Two linear equations in the same two variables are called a pair of linear equations in two variables. Standard form of linear equations in two variables.
a₁x + b₁y + c₁ = 0, a₂x + b₂y + c₁ = 0
where a₁, a₂, b₁, b₂, c₁, c₂ are real numbers such that

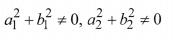
Representation of Linear Equation In Two Variables

Every linear equation in two variables graphically represents a line and each solution (x, y) of a linear equation in two variables, ax + by + c = 0, corresponds to a point on the line representing the equation, and vice versa.

Ploting Linear Equation in Two Variables on the Graph

There are infinitely many solutions of each linear equation. So, we choose at least any two values of one variable & get the value of other variable by substitution, i.e; Consider; Ax + By + C = 0 We can write the above linear equation as:

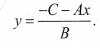
Here, we can choose any values of x & can find corresponding values of y.
After getting the values of (x, y) we plot them on the graph thereby getting the line representing Ax + By + C = 0.

Method of Solution of a Pair of Linear Equations in Two Variables

Coordinate of the point (x, y) which satisfy the system of pair of linear equations in two variables is the required solution. This is the point where the two lines representing the two equations intersect each other.
There are two methods of finding a solution of a pair of Linear equations in two variables.
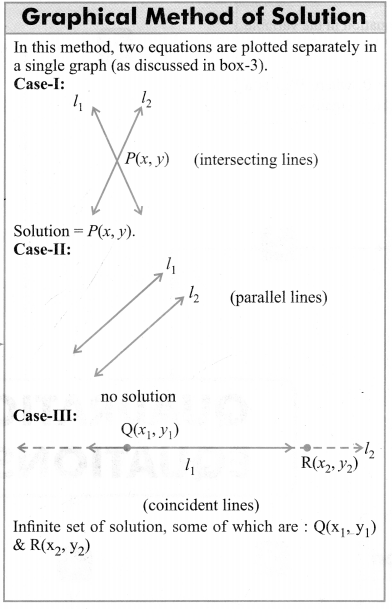
(1) Graphical Method : This method is less convenient when point representing the solution has non-integral co-ordinates.
(2) Algebraic Method : This method is more convenient when point representing the solution has non-integral co-ordinates.
This method is further divided into three methods:
(i) Substitution Method,
(ii) Elimination Method and
(iii) Cross Multiplication Method.

Consistency and Nature of the Graphs

Consider the standard form of linear equations in two variables.
a₁x + b₁y + C₁ = 0; a₂x + b₂y + c₂ = 0
While solving the above system of equation following three cases arise.
(i) If a₁a₂≠b₁b₂; system is called consistent, having unique solution and pair of straight lines representing the above equations intersect at one point only
(ii) If a₁ a₂=b₁b₂=c₁c₂ ; system is called dependent and have infinetly many solution. Pair of lines representing the equations coincide.
(iii) If a₁a₂=b₁b₂≠c₁c₂; system is called inconsistent and has no solution. Pair of lines representing the equations are parallel or do not intersect at any point.

Algebraic Method of Solution

Consider the following system of equation
a₁x + b₁y + c₁ =0; a₂x + b₂y + c₁ =0
There are following three methods under Algebraic method to solve the above system.

(i) Substitution method

(a) Find the value of one variable, say y in terms of x or x in terms of y from one equation.
(b) Substitute this value in second equation to get equation in one variable and find solution.
(c) Now substitute the value/solution so obtained in step (b) in the equation got in step (a).

(ii) Elimination Method

(a) If coefficient of any one variable are not same in both the equation multiply both the equation with suitable non-zero constants to make coefficient of any one variable numerically equal.
(b) Add or subtract the equations so obtained to get equation in one variable and solve it.
(c) Now substitute the value of the variable got in the above step in either of the original equation to get value of the other variable.

(iii) Cross multiplication method

For the pair of Linear equations intwo variables:
a₁x + b₁y + C₁ = 0
a₂x + b₂y + c₂ = 0
Consider the following diagram.

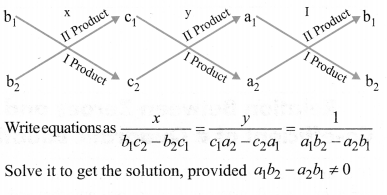
Solve it to get the solution, provided a₁b₂ – a₂b₁ ≠ 0

Equations Reducible to a Pair of Linear Equations in Two Variables

Sometimes pair of equations are not linear (or not in standard form), then they are altered so that they reduce to a pair of linear equations in standard form.
For example;

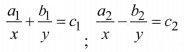
Here we substitute 1x = p & \frac{1}{y} = q, the above equations reduces to:
a₁p + b₁q = c₁ ; a₂p – b₂q = c₂
Now we can use any method to solve them.